Converting the Equation of Parabola

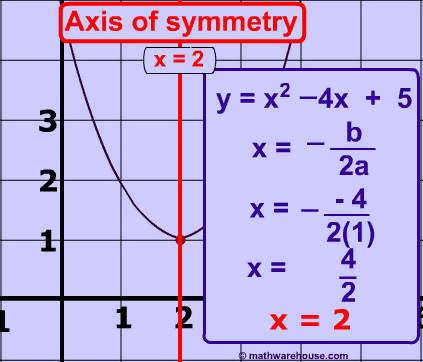
The standard form of a parabola's equation is generally expressed:

The role of a in $$ a>x^2 + bx + c $$

parabola's opens upwards like a 'U'

parabolas opens downwards like an upside down 'U'

1. If $$|a| < 1 $$, the graph of the parabola's widens. This just means that the "U" shape of parabola stretches out sideways .
2. If $$ |a| > 1 $$, the graph of the graph becomes narrower(The effect is the opposite of |a| < 1).

Axis of Symmetry in Standard Form

Vertex Form

The vertex form of a parabola's equation is generally expressed as: $$ y= a(x-h)^ 2 + k $$

• (h, k) is the vertex
• If a is positive then the parabola opens upwards like a regular "U" (same as standard form).
• If a is negative, then the graph opens downwards like an upside down "U" (same as standard form).
• If |a| < 1, the graph of the parabola widens. This just means that the "U" shape of parabola stretches out sideways.
• If |a| > 1, the graph of the graph becomes narrower (the effect is the opposite of |a| > 1).

From Vertex Form to Standard Form

From Vertex To Standard Form

Equation in vertex form: y = (x – 1)²

To convert equation to standard form simply expand and simplify the binomial square (Refresher on FOIL to multiply binomials).

Practice Problems

Problem 1

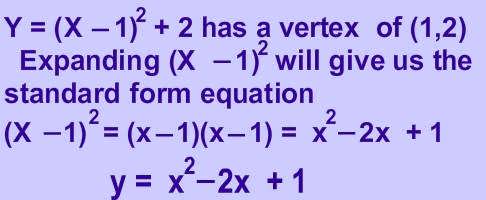
Parabola 1 has the vertex form equation: y = (x + 3)²

To rewrite this equation in standard form Expand (x+3)(x+3)

(x+3)(x+3) = x² + 3x + 3x + 9
x² + 6x + 9
y = x² + 6x + 9

Problem 2

Change the parabola's equation from vertex form to standard form. y = (x + 3)² + 4

(x+3)(x+3) + 4 = x² + 3x + 3x + 9 + 4
x² + 6x + 13
y = x² + 6x + 13

Problem 3

Change the parabola's equation from vertex form to standard form. y = (x - 3)² + 2

(x – 3)(x – 3) + 2 = x² – 3x – 3x + 9 + 2
x² – 6x + 11
y = x² – 6x + 11

Problem 4

Convert the equation below from vertex form to standard form. y - 4 = (x - 3)²

y = (x – 3)² + 4
y = x² - 6x + 9 + 4
y = x² - 6x + 13

Problem 5

Change the equation of the parabola below into standard form y - 3 = (x - 5)²

y = (x – 5)² + 3
y = x² –10x + 25 + 3
y = x² –10x + 28

Standard Form to Vertex Form

To convert an equation from standard form to vertex form it is sometimes necessary to be comfortable completing the square.

Problem 6

Convert the equation below from standard to vertex form. y = x² + 2x + 1

Problem 7

What is the vertex form of the parabola whose standard form equation is y = x² + 6x +9

Problem 8

What is the vertex form of the parabola whose standard form equation is y = x² + 6x + 10

Problem 9

Convert the equation below from standard to vertex form. y = x² + 6x + 8

Problem 10

What is the vertex form of the parabola whose standard form equation is y = x² + 10x + 25

Problem 11

What is the vertex form of the parabola whose standard form equation is y = x² + 10x + 27

(x + 5)² + 2 = (x² + 10x + 25) + 2
y = (x + 5)² + 2

Problem 12

What is the vertex form of the parabola whose standard form equation is y = x² + 10x + 21

(x + 5)² – 4 = (x² + 10x + 25) – 4
y = (x + 5)² – 4

Problem 13

Convert the equation below from standard to vertex form. y = x² + 12x + 34

(x + 6)² – 2 = (x² + 12x + 36) – 2
y = (x + 6)² – 2

Problem 14

What is the vertex form of the parabola whose standard form equation is y = x² + 14x + 40

(x + 7)² – 7 = (x² + 14x + 49) – 9
y = (x + 7)² – 9

Problem 15

Convert the equation below from standard to vertex form. y = x² + 18x + 71

(x + 9)² – 10 = (x² + 18x + 81) – 10
y = (x + 9)² – 10

Problem 16

What is the vertex form of the parabola whose standard form equation is y = x² – 16x + 71

(x – 8)² + 7 = (x² – 16x + 64) + 7
y = (x – 8)² + 7

Problem 17

What is the vertex form of the parabola whose standard form equation is y = x² + 18x + 95

(x + 9)² + 14 = (x²+ 18x + 81) + 14
y = (x + 9)² + 14

Problem 18

Convert the equation below from standard to vertex form. y = x² – 20x + 95

(x – 10)² – 5 = (x² – 20x + 100) – 5
y = (x – 10)² – 5

When "a" > 1

Practice 19

Convert the parabola's equation below to vertex form. y = 2x² + 4x + 5

2x² + 4x + 5 = 2(x² + 2x) + 5
2(x² + 2x + 1) –2 + 5
2(x² + 2x + 1) –2 + 5
2(x + 1)² +3
y = 2(x + 1)² + 3

Practice 20

Complete the square to convert the equation into vertex form. y = 2x² + 4x + 6

2x² + 4x + 6 = 2(x² + 2x) + 6
2(x² + 2x + 1) –2 + 6
2(x² + 2x + 1) –2 + 6
2(x + 1)² + 4
y = 2(x + 1)² + 4

Practice 21

Convert the parabola's equation below to vertex form. y = 3x² + 6x + 8

3x² + 6x + 8 = 3(x² + 2x) + 8
3(x² + 2x + 1) − 3 + 8
3(x + 1)² + 5
y = 3(x + 1)² + 5